Background

COMMON APPLICATIONS

Spreadsheets
Tutorial
Introduction
Worksheets
Columns & Rows
Charts

Examples
Refraction of Light
Solving Polynomial Equations
Workers' Database
Base Converter

Activities

Links

Databases
Tutorial
Introduction

Example
Periodic Table

Links

Word Processing
Tutorial
Introduction
Text
Page Properties
Printing
Quit

Examples
Address list
Time table
Newsletter
Repeat Patterns
Logos

Practical Questions

HTML Editors
Tutorial
Basic html
Netscape
Composer

Examples
Teaching Syllabus
Teachers' Notes
Subject Website Lists
Students Website

Art
Principles of Art
Elements of Art
Coloured Pencil Paintings
Pastel Paintings
Book cover
Posters


Scanning an image

Assessment objectives

By the end of chapter 2, the student should be able to:

• Derive from the definitions of velocity and acceleration, equations which represent uniformly accelerated motion in a straight line.
• Use equations of motion.
• State each of Newton's laws of motion.
• Draw force-diagrams and use them to obtain the acceleration.
• Use Newton's laws of motion in solving problems of linear motion
• State the principle of conservation of mechanical energy.
• Give examples illustrating the principle of conservation of mechanical energy.
• Apply the principle of conservation of mechanical energy in solving problems.
• State the laws of solid friction (both static and kinetic friction).
• Describe simple experiments to determine coefficients of static and kinetic friction between two solid surfaces.
• Apply the laws of solid friction in solving problems involving motion on rough surfaces.

Problems in this section involve the use of :-

1. Equations of motion.
2. Newton's laws of motion.
3. Principle of conservation of mechanical energy.
4. Laws of solid friction.




Equations of motion for uniformly accelerated rectilinear motion

The following symbols are used in this textbook:

t = time, v = final velocity, u = initial velocity

s = distance and a = acceleration.

(i) Relation between v, u, a and t .

a = ( v - u )/t

v = u + at ----------------------- (1)

(ii) Relation between s, u, t and a.

Average velocity = s/t = ( v + u )/2

s = ½(v + u ) t but v = u + at

s = ½(u + at + u )t

s = ut + ½ at² ----------------- (2)

(iii) Relation between s, v, u and a.

Combining equation (1) and equation (2)

s = u (v - u )/a + ½ a( v - u )²/a

2as = 2u (v - u) + (v - u)²

2as = v² - u²

v² = u² + 2as -------------------- (3)

(Online Resource)

Mechanics including graphs




NEWTON'S LAWS OF MOTION

Law 1: A body in its state of rest or moving in a straight line continues to do so unless acted on by an external force.

This inherent reluctance of matter to any change of motion is called inertia and law 1 may be referred to as the "Principle of inertia". The inertia of a body increases with mass.

The effects of inertia can be observed by passengers in a bus. There is a forward jerk when the vehicle stops (the motion of the passengers tending to persist ), and a backward jerk when the vehicle re-starts (the passengers tending to remain stationary).

Law 2: The rate of change of momentum of a body is proportional to the resultant force on the body and takes place in the direction of the force.

Momentum is the product of mass and velocity of a moving body.

Force = (change in momentum )/time

F = (mv - mu)/t = m(v - u)/t = ma.

F = ma

N.B: F is resultant force obtained by identifying forces acting on the system and finding the net force in the direction of motion.

Law 3: Action and reaction are equal but opposite.


Some illustrations of identification of forces and the application of Newton's laws of motion.

(i) Body of mass M placed on either a stationary platform or a platform moving at constant speed.



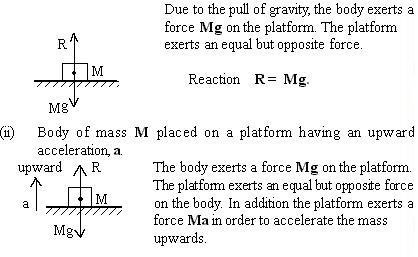
 Total force exerted by the platform = Reaction R = M(g + a)

N.B: An upward acceleration has the same effect as a downward retardation. 
 
 

---

---